A series of meetings designed to allow post-doc and Ph.D. students to present their research topics and promote collaborations.
On one Wednesday each month, a different research topic is presented in simple and accessible terms.
The seminars are designed to be attended by post-doc and Ph.D. students, but graduates and undergraduates are welcome.
The Junior Seminars are organised by Lorenzo Baroni, Rocco Brunelli, Ilaria Cruciani, Luca Ferrigno, Michele Matteucci, Martina Miseri, Mario Morellini and Federico Pieroni.
Ph.D. courses in mathematics are coordinated by Prof. Alessandro Giuliani.
Enroll yourself or visualise the events calendar.
Next event
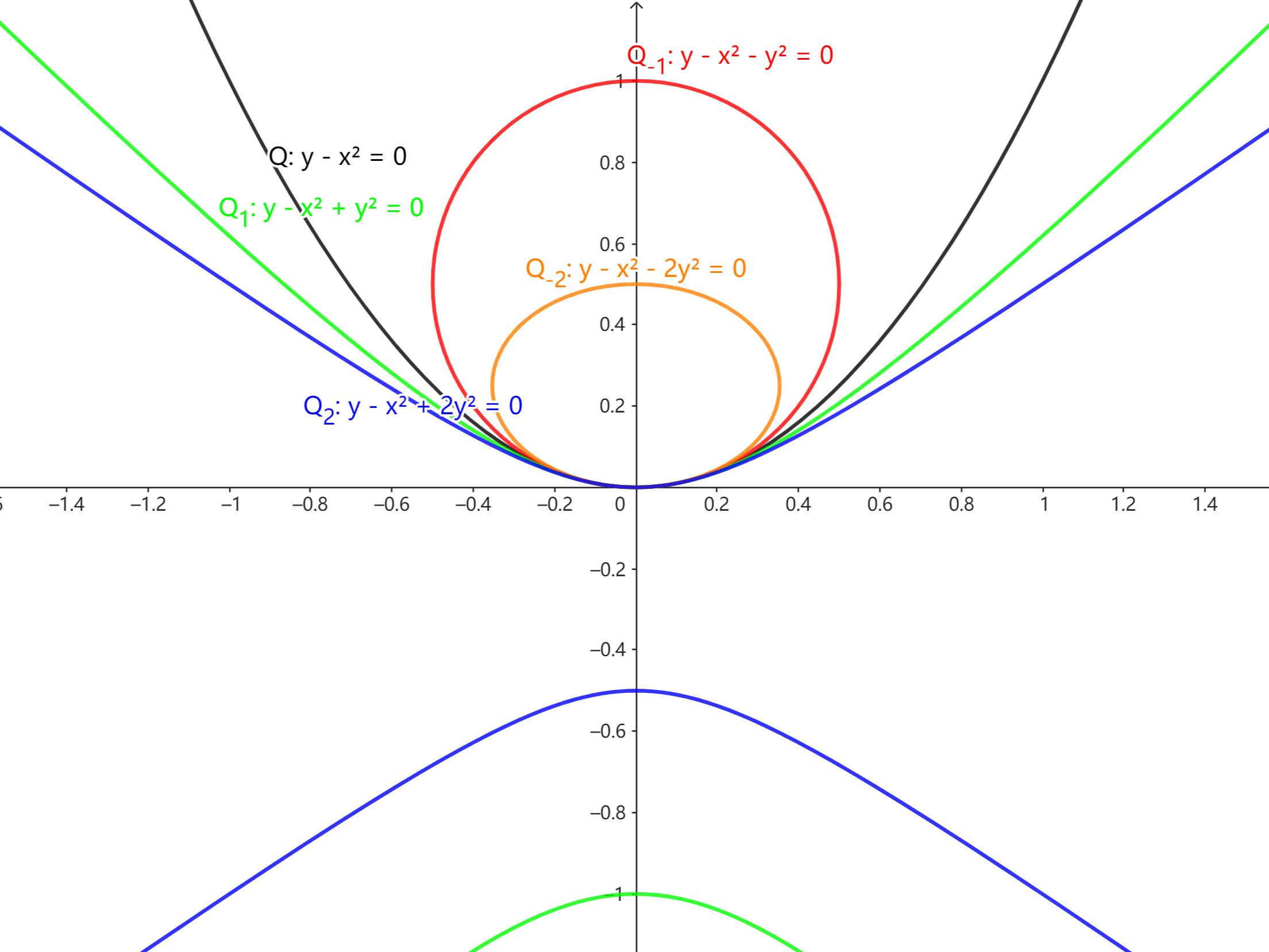
On Tangent Curves
by Wei Chen
June 25, 2025 – 16:00 – Room T.b.D.
Abstract. We begin by revisiting the notion of tangent lines to smooth plane curves from the perspective of algebraic geometry, followed by a discussion of tangent curves on the projective plane. We then introduce cusp singularities of curves and investigate the behavior of curves tangent to such singular points. Time permitting, we will also show how this type of singularity appears in recent research.
Future events
Past events
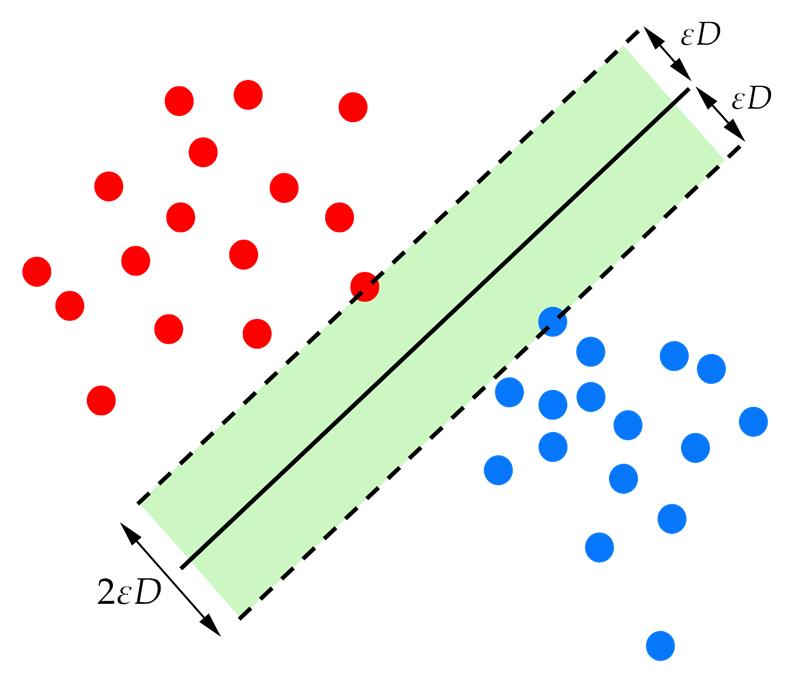
Efficient Certifying Algorithms for Linear Classification
by Sara Galatro
May 21, 2025 – 14:00 – Room B (Physics Dept.)
Abstract. This seminar explores the application of certifying algorithms to machine learning problems, namely linear separation. After introducing a few preliminary notions, we present an efficient certifying algorithm that, given a set of \(n\) real points with binary labels, either returns a hyperplane separating the points or identifies \(d+2\) of the labelled points that cannot be separated by any hyperplane using a constructive proof of Kirchberger's theorem. Finally, a dimension-free and constructive extension of Kirchberger's theorem is also discussed and applied to find \(O(1/\varepsilon^2)\) of the labelled points that cannot be separated with a normalised margin \(\varepsilon\) by any hyperplane.
Machine Learning Meets Differential Cryptanalysis
by Rocco Brunelli
April 16, 2025 – 16:00 – Room M3
Abstract.
This seminar explores the application of Machine Learning techniques in the Differential Cryptanalysis of symmetric primitives.
We introduce the fundamentals of Differential Cryptanalysis for executing differential attacks on Block Ciphers and the principles of Machine Learning for training Neural Networks. We then examine how to leverage the latter to recover key secret bits.
In the final part, we address a key challenge of these emerging techniques: the lack of generalization in feature engineering.

Hamiltonian Methods for the Kirchhoff Equation
by Simone Marrocco
March 19, 2025 – 16:00 – Room M3
Abstract.
The Kirchhoff equation with periodic boundary conditions provides a beautiful model for describing the transverse oscillations of a nonlinear elastic medium. Since its introduction in 1876, it has continued to fascinate and challenge mathematicians from all over the world.
In this presentation, we analyze its properties, highlighting both its physical motivations and mathematical implications. In particular, we discuss key theoretical questions, such as local well-posedness and the lifespan of solutions, emphasizing the challenges posed by its quasi-linear structure.
A central focus of our analysis is its Hamiltonian formulation, which provides a powerful theoretical framework for understanding the dynamics of solutions. Our goal is to offer a clear overview of the open challenges and the main techniques used to tackle them, with particular emphasis on the so-called normal form techniques.
Nonlinear Spin System
by Mario Morellini
February 26, 2025 – 14:00 – Room A (Physics Dept.)
Abstract.
In this seminar, we explore a class of nonlinear dynamics governed by the framework of mass action dynamics, which models the evolution of systems through pairwise interactions. This framework underpins several important nonlinear models across disciplines, including chemical reaction networks, Boltzmann's ideal gas model, and recombination processes in population genetics and genetic algorithms.
Our primary focus is on the nonlinear dynamics of spin systems, with an emphasis on the Ising model. Specifically, we investigate the convergence to equilibrium for spin systems at high temperatures, where non-trivial correlations are present.
Utilizing Kac's Program, we interpret these nonlinear systems as limits of Markov chain particle systems under the propagation of chaos as system size tends to infinity. This perspective enables us to estimate the rate of convergence to equilibrium. A key aspect of our approach is ensuring that the Modified Log-Sobolev inequalities used to study the particle systems remain independent of system size. When this condition is met, the nonlinear system converges to equilibrium at the same rate as its corresponding particle system.
We will present two methods for analyzing this convergence:
- A direct approach using McKean trees to study the nonlinear system.
- An indirect approach that derives convergence from particle systems using size-independent Modified Log-Sobolev inequalities.
The Prym-canonical Clifford index
by Martina Miseri
December 11, 2024 – 16:00 – Room C (Physics Dept.)
Abstract. After a brief introduction on algebraic curves and what Brill-Noether Theory is about, I will try to give an idea of the geometric meaning of the Clifford index, a classic invariant for algebraic curves. I will conclude the seminar presenting my research project, that starts with a new definition of Clifford index and focuses on the case of hyperelliptic curves.

Linear Flow on the Infinite Torus
by Lorenzo Baroni
November 20, 2024 – 16:00 – Room C (Physics Dept.)
Abstract. The celebrated Arnold-Liouville theorem provides a beautiful description of integrable Hamiltonian systems stating that the dynamics on compact invariant sets is conjugated to a linear flow on the torus \(\mathbb{T}^n := \mathbb{R}^n/\mathbb{Z}^n\). It is then clear that in trying to generalize this statement to Hamiltonian systems with infinitely many degrees of freedom, one has first to face with the issue of understanding linear flows on the infinite torus. In this talk we discuss the simple characterization occurring in finite dimension and why it fails to apply in an infinite-dimensional setting. Then, we use Pontryagin’s theory of locally compact abelian groups in order to understand the dynamics and we also discuss an analogy with the finite dimensional case, namely unique ergodicity in the absence of resonances.
Previous editions

Junior Seminars
Academic Year 2023/24
Partecipants.
- Zakaria Brahimi – Cubic fourfolds: some rational examples
- Adrien Ragot – Realisability: from constructive proofs to program specification
- Muhammad Usman – 3D Human Pose Estimation: Real-time Performance and Applications
- Federico Pieroni – An overview on Coble surfaces, their moduli, and the Coble conjecture
- Luca Ferrigno – Unlikely Intersections in diophantine geometry
- Elena Sammarco – The rationality problem for the cubic fourfold

Junior Seminars
Academic Year 2022/23
Organised by
Armando Capasso and
Elia Onofri
with the participation of
Martina Miseri and
Bruno Renzi.
Partecipants.
- Armando Capasso – What is... a Higgs Bundle?
- Elia Onofri – How machine learning can improve macroscopic models for traffic state estimation and forecast
- Simone Pesatori – Coincident root loci and the moduli space of rational elliptic surfaces
- F. C. Simon Schirren – Virtual Cycles: An Introduction
- Bruno Renzi – The dimer model: phase diagram and universality
G.T.M. – General Topics in Mathematics (informal seminars)
Academic Year 2021/22
Organised by Armando Capasso and Elia Onofri
Partecipants.
- Elia Onofri – On tweakable black-box polinomials: the cube attacks family
- Armando Capasso – Numerically flatness for Higgs bundles over compact Kähler manifolds
- Luca Ferrigno – An Overview of Diophantine Geometry
- Muhammad Usman – Symbol-based preconditioning for Riesz distributed-order space-fractional diffusion equations
- Elia Onofri – Attribute-based colouring and its applications in reducing problem's size
- Armando Capasso – Algebraic hypersurfaces with vanishing Hessian and CW-complexes
- Elia Onofri – The role of the applied mathematician: optimisation of pedestrian flows in crowded museums


